MATH108 10FA Midterm ch1-6 A
[ answers in web view ] Total points: 70

• Please show all your work! No partial credit will be given for incorrect answers with no work shown. Please draw a box around your final answer.
• Calculators are permitted, but no notes, text, laptops, PDAs, or electronic dictionaries. Cell phones should be muted and left in your pocket or bag.
• Tables 3 and 4 are attached to the back. You may detach them for your reference.

1. Classify each of the following statements (which may or may not be true) as either (D)escriptive or (I)nferential: [4]
   1. 19.2% of BC residents in 2004 were clinically obese. [(I)nferential]
   2. Of the children and adolescents studied in the 2004 Canadian Community Health Survey, 8% were clinically obese. [(D)escriptive]
   3. About 90% of students in our MATH108 class are nursing students. [(D)escriptive]
   4. 30.1% of male registered nurses (RNs) are under the age of 40. [(I)nferential]
2. Mark each of the following variables as categorical (G), ordinal (O), discrete (D), or continuous (C): [5]
   1. Whether a student passes a course or not [(G) Categorical]
   2. How many children are in a family [(D)iscrete]
   3. Satisfaction with current family doctor, rated as "Very Dissatisfied", "Dissatisfied", "Satisfied", or "Very Satisfied" [(O)rdinal]
   4. Hemoglobin count, in g/dL [(C)ontinuous]
   5. Age, divided into categories "0-10", "11-18", "19-25", "26-39", "40-59", "60 and up" [(O)rdinal]
3. Say that in a certain town 63% of the people vote Conservative, and the probability that a townsperson drives a large truck is 3/7. If you pick a random townsperson, there is a 27% chance that the townsperson is a Conservative driving a large truck.
   1. What fraction of Conservatives in this town drive large trucks? [3]
      Let C = Conservative, T = drives a large truck.
      P(T|C) = P(T and C) / P(C) = .27 / .63 = 3/7 ≈ 42.85%.
   2. In this town, is voting Conservative independent of driving a large truck? Why or why not? Interpret what this means in the context of the townspeople.[3]
      P(T|C) = 3/7 = P(T), so the two events are independent.

      In words, if we narrow our focus to Conservatives, we see that 3/7 of Conservatives drive large trucks. So we see that it doesn't matter whether a townsperson is Conservative or not, the chance of driving a large truck is still the same.
4. The diagram below is a normal probability plot for an artificially-generated dataset. (Note that the n-scores are along the horizontal axis, and the y-scores from the data are along the vertical axis; this matches the textbook.) How does the distribution differ from a normal distribution? Sketch the distribution, highlighting where and how it deviates from the normal. [5]
   
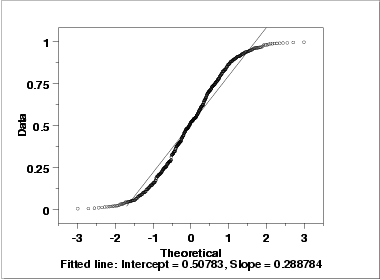
   [NIST Engineering Statistics Handbook, §1.4.2.2.2]
   [Compared with a normal distribution, both tails have been pushed inward, increasing the density in the mid-section. (The underlying distribution in this case was actually uniform, with equal probability for all values between 0 and 1.)]
5. The list below shows the total serum bilirubin level (mg/dL) in a newborn baby, measured at various times over a one-month period.
   
   4.1, 5.7, 4.7, 3.2, 4.2, 2.8, 3.6, 5.3, 4.2
   1. Construct a relative frequency histogram, classifying the data by bins of width 1 mg/dL.[4]
   2. Find the sample mean. Show your work. [2] [4.2 mg/dL]
   3. Find the sample standard deviation. Show your work. [4] [0.9381 mg/dL]
   4. Draw a boxplot for the data. Show your work. [4]
      [min=2.8, Q1=3.4, med=4.2, Q3=5.0, max=5.7]
   5. Find a 95% confidence interval for the average total serum bilirubin level for this baby over the one-month period. [4]
      [SE=0.3127, df=8, t=2.31, conf int = (3.48, 4.92)]
   6. Interpret this confidence interval in words and in the context of the study. [2]
      [We are 95% confident that the average total serum bilirubin for this baby during this period lies somewhere between 3.48 and 4.92 mg/dL.]
   7. We feel this confidence interval is still too broad. In order to estimate the average total serum bilirubin level to within ±0.4 mg/dL with 90% confidence, how many measurements would we need to average? (Assume the underlying true bilirubin level does not change.) [4]
      [z=1.65, n = 14.91, round up to 15 measurements.]
   8. If we increase the sample size to n=40 measurements, would we expect the distribution of our sample to be more normal? Why or why not? [2]
      [No, the distribution of the sample follows the distribution of the population, which does not change with sample size.]
6. An assay (test) measuring blood lactate during exercise is imprecise, returning values normally distributed around the true lactic acid concentration, with a standard deviation of 2 mmol/L. Blood lactate values of over 20 mmol/L are considered "high".
   1. What is the probability that this assay returns a result within ±2.5 mmol/L of the true value? [2]
      [z = ±2.5/2 = ±1.25, area = 78.88%]
   2. If the true concentration of blood lactate in a patient is 18.3 mmol/L (not "high"), what is the probability that the assay could still return a value that is considered "high"? [2]
      [z = (20-18.3)/2 = 0.85, area to the right = 1 - 0.8023 = 19.77%]
   3. Since the assay is so imprecise, we run it five times and average the results. Using the same true concentration of 18.3 mmol/L, what is the probability that the average of five runs is still "high"? [4]
      [SE = 2/sqrt(5) = 0.8944, z = (20-18.3)/0.8944 = 1.90, area to the right = 1 - 0.9713 = 2.87%]
7. A particular screening test for colon cancer has a 11% false-positive rate (i.e., 89% specificity) and a 7% false-negative rate (i.e., 93% sensitivity).
   1. Suppose the test is applied to a group of patients, 40% of whom are known to have colon cancer. Draw an event tree for the outcomes of the test. Label the tree with probabilities for each branch. Also calculate the probabilities of each final outcome (leaf of the tree). [5]
      [(CA,+):37.2%. (CA,-):2.8%. (noCA,+):6.6%. (noCA,-):53.4%]
   2. What is the probability that a random patient from this group will test positive for colon cancer using this screening test?[2]
      [37.2% + 6.6% = 43.8%]
   3. What is the probability that a patient from this group who tested positive actually has the disease? [2]
      [37.2% / 43.8% = 84.93%]
8. Suppose you wish to study whether male nurses are more likely than female nurses to quit within the first five years of working as a nurse.
   1. What is the population in question? [1] [Nurses (everywhere)]
   2. List the variables which need to be measured. [1]
      [Gender and whether the nurse quits within the first five years.]
   3. For each variable, indicate its level of measurement and whether it is a predictor (independent variable) or outcome (dependent variable). [2]
      [Gender: categorical/dichotomous, predictor.
      Quitting: categorical/dichotomous, outcome]
   4. Discuss how you might do the sampling process. What are some steps you could take to ensure a random sampling? Address both criteria for random sampling. How would you measure the variables? Would the measurement process bias your sampling? [3]
      [Employment records from hospitals/clinics: find all nurses who quit within five years, and all nurses who have been working as nurses for over five years. (Don't include nurses who have been working less than five years but haven't quit.)]